Kirchhoff’s Laws are fundamental principles in electrical engineering and physics that describe the behavior of electric circuits. Formulated by the German physicist Gustav Kirchhoff in the mid-19th century, these laws provide essential tools for analyzing complex electrical networks. This extensive article will delve into the two primary laws—Kirchhoff’s Current Law (KCL) and Kirchhoff’s Voltage Law (KVL)—their mathematical formulations, applications, and illustrative explanations of each concept.
Overview of Kirchhoff’s Laws
Kirchhoff’s Laws consist of two key principles that govern the flow of electric current and the distribution of voltage in electrical circuits:
1. Kirchhoff’s Current Law (KCL): This law deals with the conservation of electric charge in a circuit.
2. Kirchhoff’s Voltage Law (KVL): This law addresses the conservation of energy in electrical circuits.
Together, these laws form the foundation for circuit analysis and are widely used in both theoretical and practical applications.
Kirchhoff’s Current Law (KCL)
Definition
Kirchhoff’s Current Law states that the total current entering a junction (or node) in an electrical circuit is equal to the total current leaving that junction. This principle is based on the conservation of electric charge, which implies that charge cannot accumulate at a junction.
Mathematical Formulation
Mathematically, KCL can be expressed as:
![\[ \sum I_{\text{in}} = \sum I_{\text{out}} \]](https://pengayaan.com/blog/wp-content/uploads/2024/12/quicklatex.com-3794c52de5685f26b32861a8acb652bb_l3.png "Rendered by QuickLaTeX.com")
Where:
is the sum of currents flowing into the junction.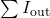
is the sum of currents flowing out of the junction.
Illustrative Explanation
Imagine a busy intersection where several roads converge. The cars entering the intersection represent currents flowing into the junction, while the cars leaving the intersection represent currents flowing out. According to KCL, the number of cars entering the intersection must equal the number of cars leaving it, assuming no cars are parked or stopped at the intersection. This analogy illustrates the principle of charge conservation at a junction in an electrical circuit.
Application of KCL
KCL is used extensively in circuit analysis to determine unknown currents in complex networks. For example, consider a junction where three currents meet: 
enters the junction, and 
and 
leave the junction. According to KCL:
![\[ I_1 = I_2 + I_3 \]](https://pengayaan.com/blog/wp-content/uploads/2024/12/quicklatex.com-584d9954516349e5c5c7546b9cd43ba3_l3.png "Rendered by QuickLaTeX.com")
Substituting the known values:
![\[ 5 \, \text{A} = 3 \, \text{A} + I_3 \]](https://pengayaan.com/blog/wp-content/uploads/2024/12/quicklatex.com-c0421afb42d0d5e267fdea24313bfcf2_l3.png "Rendered by QuickLaTeX.com")
Solving for :
![\[ I_3 = 5 \, \text{A} - 3 \, \text{A} = 2 \, \text{A} \]](https://pengayaan.com/blog/wp-content/uploads/2024/12/quicklatex.com-f126755c52d914671f7a023cc9f3ae15_l3.png "Rendered by QuickLaTeX.com")
This example demonstrates how KCL can be applied to find unknown currents in a circuit.
Kirchhoff’s Voltage Law (KVL)
Definition
Kirchhoff’s Voltage Law states that the sum of the electrical potential differences (voltages) around any closed loop in a circuit is equal to zero. This principle is based on the conservation of energy, which implies that the energy supplied by sources (like batteries) must equal the energy consumed by components (like resistors) in the loop.
Mathematical Formulation
Mathematically, KVL can be expressed as:
![\[ \sum V = 0 \]](https://pengayaan.com/blog/wp-content/uploads/2024/12/quicklatex.com-145840147b03b49737eac2f64423545e_l3.png "Rendered by QuickLaTeX.com")
Where:
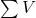is the algebraic sum of all voltages in a closed loop.
Illustrative Explanation
Consider a circular track where runners represent electrical energy. If one runner starts at a point and runs around the track, they must return to the starting point with the same amount of energy they had when they began. If they gain energy from a water station (voltage source) and lose energy by running uphill (voltage drop across a resistor), the total energy gained and lost must balance out to zero. This analogy illustrates the principle of energy conservation in a closed loop of an electrical circuit.
Application of KVL
KVL is used to analyze circuits by writing equations that relate the voltages across various components in a loop. For example, consider a simple loop with a battery (voltage 
) and two resistors (
and 
) with voltage drops 
and 
respectively. According to KVL:
![\[ V - V_1 - V_2 = 0 \]](https://pengayaan.com/blog/wp-content/uploads/2024/12/quicklatex.com-4ed80388ddf1e098414b8024489b92f7_l3.png "Rendered by QuickLaTeX.com")
Rearranging gives:
![\[ V = V_1 + V_2 \]](https://pengayaan.com/blog/wp-content/uploads/2024/12/quicklatex.com-e1d1d38d6d562c63cf71716f13dc44b7_l3.png "Rendered by QuickLaTeX.com")
If 
, 
, we can find :
![\[ 12 \, \text{V} = 5 \, \text{V} + V_2 \implies V_2 = 12 \, \text{V} - 5 \, \text{V} = 7 \, \text{V} \]](https://pengayaan.com/blog/wp-content/uploads/2024/12/quicklatex.com-d79b8bfa2a1708f7699ff8153d538d14_l3.png "Rendered by QuickLaTeX.com")
This example demonstrates how KVL can be applied to find unknown voltages in a circuit.
Applications of Kirchhoff’s Laws
Kirchhoff’s Laws are widely used in various applications, including:
1. Circuit Analysis: KCL and KVL are fundamental tools for analyzing complex electrical circuits, allowing engineers to determine unknown currents and voltages.
Illustrative Explanation
When designing a circuit, engineers can use KCL and KVL to ensure that the circuit functions correctly, just as an architect uses principles of balance and symmetry to design a stable building.
2. Electrical Engineering: These laws are essential in the design and analysis of electrical systems, including power distribution networks, communication systems, and electronic devices.
Illustrative Explanation
In a power distribution network, KCL helps ensure that the current supplied to various branches of the network is balanced, preventing overloads and ensuring efficient operation.
3. Educational Purposes: Kirchhoff’s Laws are fundamental concepts taught in physics and electrical engineering courses, helping students understand the principles of circuit behavior.
Illustrative Explanation
Students often conduct experiments in laboratories to observe KCL and KVL in action, reinforcing their understanding of these fundamental principles.
4. Troubleshooting Circuits: Technicians use Kirchhoff’s Laws to diagnose issues in electrical circuits by analyzing current and voltage distributions.
Illustrative Explanation
When a circuit malfunctions, technicians can apply KCL and KVL to identify where the problem lies, similar to how a mechanic uses diagnostic tools to pinpoint issues in a car.
Conclusion
In conclusion, Kirchhoff’s Laws are essential principles in electrical engineering and physics that describe the behavior of electric circuits. Kirchhoff’s Current Law (KCL) emphasizes the conservation of electric charge at junctions, while Kirchhoff’s Voltage Law (KVL) highlights the conservation of energy in closed loops. Together, these laws provide a framework for analyzing and understanding complex electrical networks, making them invaluable tools for engineers, technicians, and students alike. By grasping these concepts, one can gain a deeper appreciation for the intricate workings of electrical systems and their applications in our daily lives.