Mole Fraction: A Comprehensive Exploration

Mole fraction is a fundamental concept in chemistry that quantifies the concentration of a component in a mixture. It is particularly useful in the study of solutions, gases, and various chemical reactions. The mole fraction provides a way to express the relative amount of a substance in relation to the total amount of all substances present in a mixture. This article aims to provide an exhaustive overview of mole fraction, including its definition, calculation methods, significance, applications, and illustrative explanations of each concept.

Definition of Mole Fraction

The mole fraction ( $X$ ) of a component in a mixture is defined as the ratio of the number of moles of that component to the total number of moles of all components in the mixture. Mathematically, it can be expressed as:

$X_i = \frac{n_i}{n_{\text{total}}}$

Where:

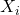$X_i$ is the mole fraction of component $i$ .
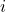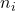$n_i$ is the number of moles of component $i$ .
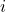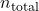$n_{\text{total}}$ is the total number of moles of all components in the mixture.

Illustrative Explanation: Consider a mixture of gases containing 2 moles of oxygen ( $O_2$ ) and 3 moles of nitrogen ( $N_2$ ). The total number of moles in the mixture is:

$n_{\text{total}} = n_{O_2} + n_{N_2} = 2 + 3 = 5 \text{ moles}$

The mole fraction of oxygen can be calculated as:

$X_{O_2} = \frac{n_{O_2}}{n_{\text{total}}} = \frac{2}{5} = 0.4$

Similarly, the mole fraction of nitrogen is:

$X_{N_2} = \frac{n_{N_2}}{n_{\text{total}}} = \frac{3}{5} = 0.6$

Properties of Mole Fraction

1. Dimensionless Quantity:

Mole fraction is a dimensionless quantity, meaning it has no units. It is expressed as a ratio and can take values between 0 and 1.

Illustrative Explanation: A mole fraction of 0 indicates that the component is absent from the mixture, while a mole fraction of 1 indicates that the component constitutes the entire mixture.

2. Sum of Mole Fractions:

The sum of the mole fractions of all components in a mixture is always equal to 1.

$\sum X_i = 1$

Illustrative Example: In the previous example, the sum of the mole fractions of oxygen and nitrogen is:

$X_{O_2} + X_{N_2} = 0.4 + 0.6 = 1$

3. Independent of Temperature and Pressure:

Mole fraction is independent of temperature and pressure, making it a useful measure in various conditions, especially in gas mixtures.

Illustrative Explanation: Whether the gas mixture is at high pressure or low pressure, the mole fraction remains the same as long as the composition of the mixture does not change.

Calculation of Mole Fraction

To calculate the mole fraction of a component in a mixture, follow these steps:

1. Determine the Number of Moles:

Calculate the number of moles of each component using the formula:

$n = \frac{m}{M}$

Where:

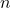$n$ is the number of moles.
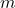$m$ is the mass of the component (in grams).
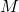$M$ is the molar mass of the component (in g/mol).

Illustrative Example: If you have 10 grams of water ( $H_2O$ ) and the molar mass of water is approximately 18 g/mol, the number of moles of water is:

$n_{H_2O} = \frac{10 \, \text{g}}{18 \, \text{g/mol}} \approx 0.56 \, \text{moles}$

2. Calculate the Total Number of Moles:

Add the number of moles of all components in the mixture.

Illustrative Explanation: If you also have 5 grams of sodium chloride ( $NaCl$ ) with a molar mass of approximately 58.5 g/mol, the number of moles of sodium chloride is:

$n_{NaCl} = \frac{5 \, \text{g}}{58.5 \, \text{g/mol}} \approx 0.085 \, \text{moles}$

The total number of moles is:

$n_{\text{total}} = n_{H_2O} + n_{NaCl} \approx 0.56 + 0.085 \approx 0.645 \, \text{moles}$

3. Calculate the Mole Fraction:

Use the mole fraction formula to find the mole fraction of each component.

Illustrative Example: The mole fraction of water is:

$X_{H_2O} = \frac{n_{H_2O}}{n_{\text{total}}} \approx \frac{0.56}{0.645} \approx 0.87$

The mole fraction of sodium chloride is:

$X_{NaCl} = \frac{n_{NaCl}}{n_{\text{total}}} \approx \frac{0.085}{0.645} \approx 0.13$

Significance of Mole Fraction

Mole fraction is significant in various scientific and engineering contexts:

1. Chemical Reactions:

In chemical reactions, mole fractions are used to express the composition of reactants and products. They are essential for stoichiometric calculations and determining reaction yields.

Illustrative Explanation: In a reaction where 2 moles of hydrogen react with 1 mole of oxygen to produce water, knowing the mole fractions of the reactants helps predict how much product will be formed.

2. Colligative Properties:

Mole fraction is used to calculate colligative properties, such as boiling point elevation and freezing point depression, which depend on the number of solute particles in a solution.

Illustrative Example: When salt is added to water, the mole fraction of water decreases, leading to a lower freezing point and a higher boiling point compared to pure water.

3. Gas Mixtures:

In gas mixtures, mole fraction is used to determine partial pressures and concentrations of individual gases. According to Dalton’s Law of Partial Pressures, the total pressure of a gas mixture is the sum of the partial pressures of each gas.

Illustrative Explanation: If a mixture contains 3 moles of oxygen and 2 moles of nitrogen, the partial pressure of oxygen can be calculated using its mole fraction and the total pressure of the mixture.

4. Thermodynamics:

Mole fraction is important in thermodynamics, particularly in calculating the Gibbs free energy and chemical potential of components in a mixture.

Illustrative Example: In a solution, the chemical potential of a solute is related to its mole fraction, influencing the direction of spontaneous processes.

Applications of Mole Fraction

Mole fraction has numerous applications across various fields:

1. Pharmaceuticals:

In drug formulation, mole fraction is used to determine the concentration of active ingredients in a solution, ensuring proper dosing and efficacy.

Illustrative Explanation: A pharmaceutical solution may require a specific mole fraction of an active ingredient to achieve the desired therapeutic effect.

2. Environmental Science:

Mole fraction is used in environmental studies to analyze the composition of pollutants in air and water, helping assess environmental impact and compliance with regulations.

Illustrative Example: Monitoring the mole fraction of carbon dioxide in the atmosphere is crucial for understanding climate change and its effects.

3. Material Science:

In material science, mole fraction is used to study the properties of alloys and composite materials, influencing their mechanical and thermal characteristics.

Illustrative Explanation: The performance of a metal alloy can be optimized by adjusting the mole fractions of its constituent elements.

4. Food Science:

In food science, mole fraction is used to analyze the composition of food products, including flavor compounds and preservatives, ensuring safety and quality.

Illustrative Example: The mole fraction of sugar in a beverage affects its sweetness and overall flavor profile.

Conclusion

Mole fraction is a fundamental concept in chemistry that provides a quantitative measure of the concentration of components in a mixture. By defining the ratio of the number of moles of a component to the total number of moles, mole fraction serves as a crucial tool for understanding chemical reactions, colligative properties, gas mixtures, and thermodynamic processes. Its significance extends across various fields, including pharmaceuticals, environmental science, material science, and food science. By grasping the concept of mole fraction and its applications, we can better analyze and interpret the behavior of mixtures in the natural world. As we continue to explore the principles of chemistry, mole fraction remains a key concept that underpins our understanding of the composition and behavior of substances. Recognizing the importance of mole fraction not only enhances our comprehension of chemical systems but also informs the development of technologies and practices that impact our daily lives.

Blog.Pengayaan.Com