Biot-savart Law – Blog.Pengayaan.Com

The Biot-Savart Law is a fundamental principle in electromagnetism that describes the magnetic field generated by an electric current. Named after French physicists Jean-Baptiste Biot and Félix Savart, who formulated the law in the early 19th century, it provides a mathematical framework for understanding how currents produce magnetic fields in their vicinity. This article aims to provide a comprehensive understanding of the Biot-Savart Law, including its formulation, derivation, applications, and significance in both theoretical and practical contexts.

Historical Background

The Biot-Savart Law emerged from the early studies of electricity and magnetism, which were rapidly evolving fields in the 18th and 19th centuries. The relationship between electric currents and magnetic fields was first observed by Hans Christian Ørsted in 1820, who discovered that a current-carrying wire could deflect a nearby compass needle, indicating the presence of a magnetic field.

Building on Ørsted’s findings, Biot and Savart conducted experiments to quantify the relationship between electric currents and the resulting magnetic fields. Their work culminated in the formulation of the Biot-Savart Law, which mathematically describes how the magnetic field ( $\mathbf{B}$ ) at a point in space is influenced by the current flowing through a conductor.

The Biot-Savart Law: Formulation

The Biot-Savart Law states that the magnetic field $\mathbf{B}$ at a point in space due to a small segment of current-carrying wire is directly proportional to the current ( $I$ ) flowing through the wire and inversely proportional to the square of the distance ( $r$ ) from the wire to the point where the magnetic field is being measured. The law can be mathematically expressed as:

$\mathbf{B} = \frac{\mu_0}{4\pi} \int \frac{I \, d\mathbf{l} \times \hat{\mathbf{r}}}{r^2}$

Where:

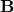$\mathbf{B}$ is the magnetic field vector at the point of interest,
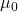$\mu_0$ is the permeability of free space (approximately $4\pi \times 10^{-7} \, \text{T m/A}$ ),
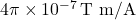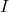$I$ is the current flowing through the wire,
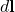$d\mathbf{l}$ is a differential length vector of the current element,
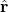$\hat{\mathbf{r}}$ is the unit vector pointing from the current element to the point where the magnetic field is being calculated,
$r$ is the distance from the current element to the point of interest.

Derivation of the Biot-Savart Law

The derivation of the Biot-Savart Law involves several key concepts from vector calculus and electromagnetism. The following steps outline the derivation:

1. Magnetic Field Contribution: Consider a small segment of wire carrying a current $I$ . The magnetic field $d\mathbf{B}$ produced by this segment at a point in space is proportional to the current and the length of the segment $d\mathbf{l}$ .

2. Cross Product: The direction of the magnetic field is determined by the right-hand rule, which states that if the thumb of the right hand points in the direction of the current, the curled fingers point in the direction of the magnetic field. This relationship is captured by the cross product $d\mathbf{l} \times \hat{\mathbf{r}}$ .

3. Distance Factor: The strength of the magnetic field decreases with the square of the distance from the current element, leading to the $\frac{1}{r^2}$ term in the equation.

4. Integration: To find the total magnetic field $\mathbf{B}$ at a point due to the entire current-carrying wire, the contributions from all segments of the wire must be integrated.

The result of this derivation is the Biot-Savart Law, which provides a powerful tool for calculating magnetic fields generated by various current configurations.

Applications of the Biot-Savart Law

The Biot-Savart Law has numerous applications in both theoretical and practical contexts:

1. Magnetic Field Calculation: The law is used to calculate the magnetic field produced by various current configurations, including straight wires, loops, and solenoids. For example, the magnetic field at the center of a circular loop of wire can be derived using the Biot-Savart Law.

2. Electromagnetic Devices: The Biot-Savart Law is fundamental in the design and analysis of electromagnetic devices such as transformers, inductors, and electric motors. Understanding the magnetic fields generated by currents is essential for optimizing the performance of these devices.

3. Magnetic Field Mapping: The law is employed in experimental physics to map the magnetic fields produced by current-carrying conductors. This mapping is crucial for understanding the behavior of magnetic fields in various applications, including magnetic resonance imaging (MRI) and particle accelerators.

4. Electromagnetic Theory: The Biot-Savart Law is a cornerstone of classical electromagnetism and is often used in conjunction with Ampère’s Law and Faraday’s Law of Induction to analyze complex electromagnetic systems.

5. Astrophysics and Geophysics: The principles underlying the Biot-Savart Law are applied in astrophysics to study the magnetic fields of celestial bodies and in geophysics to understand the Earth’s magnetic field and its variations.

Limitations of the Biot-Savart Law

While the Biot-Savart Law is a powerful tool for calculating magnetic fields, it has certain limitations:

1. Static Currents: The Biot-Savart Law is applicable primarily to static or steady currents. It does not account for time-varying currents, which require the use of Maxwell’s equations for a complete description of electromagnetic fields.

2. Complex Geometries: For highly complex current distributions, the integration required to apply the Biot-Savart Law can become mathematically challenging. In such cases, numerical methods or computational techniques may be necessary.

3. Non-Uniform Media: The law assumes a uniform medium (vacuum or air) around the current-carrying conductor. In cases where the medium is non-uniform or has magnetic properties, additional considerations must be taken into account.

Conclusion

The Biot-Savart Law is a fundamental principle in electromagnetism that describes the relationship between electric currents and the magnetic fields they produce. Its formulation, derivation, and applications provide valuable insights into the behavior of magnetic fields in various contexts, from simple circuits to complex electromagnetic devices. Understanding the Biot-Savart Law is essential for students and professionals in physics and engineering, as it forms the basis for many concepts in electromagnetism and plays a critical role in the design and analysis of electrical systems. As technology continues to advance, the principles encapsulated in the Biot-Savart Law will remain integral to the development of innovative solutions in electronics, energy systems, and beyond.

Blog.Pengayaan.Com