The Hall Effect is a fundamental phenomenon in electromagnetism that describes the behavior of charged particles in a magnetic field. Discovered by American physicist Edwin Hall in 1879, this effect has significant implications in various fields, including physics, engineering, and materials science. This article will delve into the definition of the Hall Effect, its underlying principles, mathematical formulation, experimental setup, applications, and illustrative explanations for each concept.
Definition of the Hall Effect
The Hall Effect refers to the generation of a voltage difference (the Hall voltage) across a conductor or semiconductor when it is placed in a magnetic field perpendicular to the direction of the electric current flowing through it. This voltage arises due to the Lorentz force acting on the charge carriers (electrons or holes) within the material, causing them to accumulate on one side of the conductor, leading to a measurable voltage across the material.
Illustrative Explanation
To visualize the Hall Effect, imagine a river (the electric current) flowing through a valley (the conductor). If a strong wind (the magnetic field) blows across the valley at a right angle to the river, it pushes the water (the charge carriers) to one side. As a result, the water accumulates on one bank of the valley, creating a difference in water level (the Hall voltage) between the two sides. This analogy helps illustrate how the interaction between the current and the magnetic field leads to the generation of a voltage difference.
Underlying Principles of the Hall Effect
The Hall Effect is based on several key principles of electromagnetism:
1. Lorentz Force: The Lorentz force is the force experienced by a charged particle moving through a magnetic field. It is given by the equation:
![\[ \mathbf{F} = q(\mathbf{v} \times \mathbf{B}) \]](https://pengayaan.com/blog/wp-content/uploads/2024/12/quicklatex.com-18da3c71ca6963c32ae35763b708ede4_l3.png "Rendered by QuickLaTeX.com")
Where:
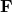is the force acting on the charge.
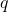is the charge of the particle.
is the velocity of the particle.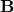
is the magnetic field.
The direction of the force is perpendicular to both the velocity of the charge and the magnetic field, leading to the deflection of charge carriers.
Illustrative Explanation
Imagine a baseball (the charged particle) being thrown straight down a field (the conductor). If a strong wind (the magnetic field) blows across the field, the baseball will be pushed sideways. The direction of the push depends on the direction of the wind and the throw, illustrating how the Lorentz force acts on moving charges.
2. Charge Carrier Accumulation: When the charge carriers are deflected by the Lorentz force, they accumulate on one side of the conductor, creating a charge imbalance. This accumulation leads to the development of an electric field across the material, which opposes further accumulation of charge carriers.
Illustrative Explanation
Think of a crowd of people (charge carriers) trying to walk through a narrow hallway (the conductor). If someone pushes from the side (the magnetic field), people will start to gather on one side of the hallway. As more people accumulate, they create a barrier that prevents others from moving to that side, similar to how the electric field develops to counteract further charge accumulation.
3. Hall Voltage: The voltage difference created across the conductor due to the accumulation of charge carriers is known as the Hall voltage (
). It can be measured and is directly proportional to the magnetic field strength and the current flowing through the conductor.
Illustrative Explanation
Imagine a water tank with two sides. As water accumulates on one side due to the wind (magnetic field), the height of the water on that side increases. The difference in height between the two sides represents the Hall voltage, which can be measured to understand the effect of the wind on the water flow.
Mathematical Formulation of the Hall Effect
The Hall voltage can be quantitatively expressed using the following formula:
![\[ V_H = \frac{B \cdot I}{n \cdot e \cdot d} \]](https://pengayaan.com/blog/wp-content/uploads/2024/12/quicklatex.com-d3520cfcb270287b4e09f0eb9512c556_l3.png "Rendered by QuickLaTeX.com")
Where:
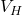- is the Hall voltage.
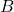is the magnetic field strength.
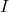is the current flowing through the conductor.
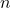is the charge carrier density (number of charge carriers per unit volume).
is the elementary charge (approximately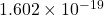 
coulombs).
is the thickness of the conductor.
Illustrative Explanation
To understand this formula, consider a simple analogy of a water pipe. The flow of water (current) through the pipe is influenced by the pressure (magnetic field) and the size of the pipe (thickness). If you increase the pressure or the flow rate, the height of the water in a connected tank (Hall voltage) will rise. Similarly, the Hall voltage is influenced by the magnetic field, current, charge carrier density, and thickness of the conductor.
Experimental Setup for the Hall Effect
To observe the Hall Effect, a typical experimental setup includes the following components:
1. Conductor or Semiconductor Sample: A thin strip of material (metal or semiconductor) is used to conduct the electric current.
2. Current Source: A power supply is connected to the sample to provide a steady electric current.
3. Magnetic Field Source: An external magnet or electromagnet is used to create a magnetic field perpendicular to the current flow.
4. Voltmeter: A voltmeter is connected across the width of the sample to measure the Hall voltage generated.
Illustrative Explanation
Imagine setting up a small water channel (the conductor) with a pump (current source) to push water through it. You then place a fan (magnetic field source) blowing across the channel. As the water flows, you can measure the height difference on either side of the channel (Hall voltage) using a ruler (voltmeter). This setup allows you to observe how the interaction between the water flow and the wind creates a measurable effect.
Applications of the Hall Effect
The Hall Effect has numerous practical applications across various fields:
1. Magnetic Field Sensors: Hall Effect sensors are widely used to measure magnetic fields in various applications, including automotive systems, industrial equipment, and consumer electronics. These sensors can detect the presence and strength of magnetic fields, enabling functionalities such as position sensing and speed detection.
Illustrative Explanation
Think of a traffic light that changes color based on the presence of cars. A Hall Effect sensor can detect the magnetic field generated by the car’s engine, allowing the traffic light to respond accordingly. This is similar to how Hall Effect sensors work in various devices.
2. Current Sensors: Hall Effect devices can measure electric current without direct contact with the conductor. By detecting the magnetic field generated by the current, these sensors provide a non-invasive way to monitor current flow in electrical circuits.
Illustrative Explanation
Imagine a security camera that can detect movement without physically touching anything. A Hall Effect current sensor works similarly, sensing the magnetic field around a wire to determine how much current is flowing without needing to connect directly to the wire.
3. Magnetic Levitation: The Hall Effect is utilized in magnetic levitation systems, such as maglev trains. These trains use powerful magnets to lift and propel the train above the tracks, reducing friction and allowing for high-speed travel.
Illustrative Explanation
Picture a hovercraft gliding above the water’s surface. The Hall Effect helps create a similar effect in maglev trains, where powerful magnets lift the train off the tracks, allowing it to move smoothly and quickly without touching the ground.
4. Semiconductor Characterization: The Hall Effect is used to determine the type and concentration of charge carriers in semiconductors. By measuring the Hall voltage, researchers can gain insights into the electrical properties of materials, which is crucial for developing electronic devices.
Illustrative Explanation
Think of a scientist analyzing the ingredients in a recipe. By measuring the Hall voltage, they can determine the “ingredients” (charge carriers) in a semiconductor, helping them understand how to create better electronic components.
Conclusion
In conclusion, the Hall Effect is a fundamental phenomenon that describes the generation of a voltage difference across a conductor or semiconductor when placed in a magnetic field perpendicular to the electric current. This effect arises from the Lorentz force acting on charge carriers, leading to their accumulation on one side of the material and the development of a measurable Hall voltage. The Hall Effect has numerous practical applications, including magnetic field sensors, current sensors, magnetic levitation systems, and semiconductor characterization. By understanding the principles and implications of the Hall Effect, we can appreciate its significance in both scientific research and technological advancements.