Kinetic Theory of Gases: A Comprehensive Exploration of Its Principles, Assumptions, and Applications

The kinetic theory of gases is a fundamental scientific theory that explains the behavior of gases in terms of the motion of their constituent molecules. This theory provides a molecular-level understanding of gas properties, such as pressure, temperature, and volume, and is essential for explaining various phenomena in physics and chemistry. By considering gases as collections of particles in constant motion, the kinetic theory offers insights into the macroscopic properties of gases based on microscopic behavior. This article aims to provide an exhaustive overview of the kinetic theory of gases, detailing its principles, assumptions, mathematical formulations, and applications, along with illustrative explanations of each concept.

Understanding the Kinetic Theory of Gases

1. Basic Principles of the Kinetic Theory

The kinetic theory of gases is based on several key principles that describe the behavior of gas molecules:

a. Molecular Motion

Gas molecules are in constant, random motion. They move in straight lines until they collide with other molecules or the walls of their container. This motion is influenced by temperature, with higher temperatures corresponding to greater molecular speeds.

• Illustrative Explanation: Imagine a crowded room filled with people moving around. Each person represents a gas molecule, and they move in various directions, occasionally bumping into each other or the walls of the room. The faster they move, the more crowded the room feels, similar to how gas molecules behave at higher temperatures.

b. Elastic Collisions

Collisions between gas molecules and between molecules and the walls of the container are perfectly elastic. This means that there is no loss of kinetic energy during collisions; instead, the energy is transferred between the colliding particles.

• Illustrative Explanation: Think of a game of billiards. When the cue ball strikes another ball, the collision is elastic; the balls bounce off each other without losing energy. Similarly, gas molecules collide elastically, maintaining their total kinetic energy.

c. Negligible Intermolecular Forces

In an ideal gas, the intermolecular forces between gas molecules are negligible. This assumption simplifies the analysis, as it allows us to treat gas molecules as independent entities that do not attract or repel each other significantly.

• Illustrative Explanation: Picture a group of friends at a party, each standing far apart and not interacting with one another. They can move freely without affecting each other’s motion, just like gas molecules in an ideal gas.

2. Assumptions of the Kinetic Theory

The kinetic theory of gases is built on several assumptions that help simplify the analysis of gas behavior:

a. Large Number of Molecules

The theory assumes that a gas consists of a large number of molecules, which allows for statistical analysis. The behavior of individual molecules is less important than the average behavior of the entire gas.

• Illustrative Explanation: Imagine a jar filled with thousands of marbles. While each marble can move independently, it is more useful to consider the overall behavior of all the marbles together rather than focusing on individual movements.

b. Random Distribution of Velocities

The velocities of gas molecules are randomly distributed, following a statistical distribution known as the Maxwell-Boltzmann distribution. This distribution describes the range of speeds that gas molecules can have at a given temperature.

• Illustrative Explanation: Think of a race where runners have different speeds. Some runners are fast, while others are slow. The overall performance of the group can be described by the average speed, but individual speeds vary widely, similar to how gas molecules have a range of velocities.

c. Volume of Molecules is Negligible

The volume of individual gas molecules is negligible compared to the volume of the container. This assumption allows us to treat gas molecules as point particles, simplifying calculations.

• Illustrative Explanation: Imagine a balloon filled with air. The air molecules inside are tiny compared to the size of the balloon. For practical purposes, we can ignore the size of the individual molecules and focus on the overall volume of the gas.

Mathematical Formulation of the Kinetic Theory

3. Pressure and Kinetic Theory

One of the key insights of the kinetic theory of gases is the relationship between molecular motion and pressure. Pressure () exerted by a gas on the walls of its container can be derived from the kinetic theory as follows:

a. Derivation of Pressure

The pressure exerted by a gas is the result of collisions between gas molecules and the walls of the container. The force exerted by these collisions is related to the change in momentum of the molecules. The formula for pressure can be expressed as:

   

Where:

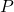• is the pressure,
• is the number of molecules,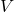
• is the volume of the container,
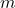• is the mass of a gas molecule,
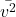• is the average of the square of the molecular speeds.
• Illustrative Explanation: Imagine a basketball being bounced against a wall. Each time the ball hits the wall, it exerts a force. The more times the ball hits the wall in a given time, the greater the pressure it exerts. Similarly, gas molecules collide with the walls of their container, creating pressure based on their speed and frequency of collisions.

4. Temperature and Kinetic Energy

The kinetic theory also establishes a direct relationship between the temperature of a gas and the average kinetic energy of its molecules. The average kinetic energy () of a gas molecule can be expressed as:

   

Where:

• is the average kinetic energy,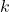
• is the Boltzmann constant (),
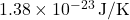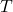• is the absolute temperature in Kelvin.
• Illustrative Explanation: Consider a group of children playing on a playground. On a warm day, they run around energetically (high kinetic energy), while on a cold day, they move slowly (low kinetic energy). The temperature of the playground corresponds to the average kinetic energy of the children, just as the temperature of a gas relates to the average kinetic energy of its molecules.

Applications of the Kinetic Theory of Gases

5. Ideal Gas Law

The kinetic theory of gases provides the foundation for the ideal gas law, which relates pressure, volume, temperature, and the number of moles of a gas. The ideal gas law is expressed as:

   

Where:

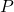• is the pressure,
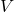• is the volume,
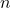• is the number of moles,
• is the ideal gas constant (),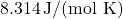
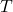• is the absolute temperature.
• Illustrative Explanation: Imagine a balloon filled with air. If you increase the temperature (heat it), the air molecules inside move faster, increasing the pressure against the walls of the balloon. The ideal gas law helps us understand how changes in temperature, volume, and the number of moles of gas affect the pressure.

6. Real Gases and Deviations from Ideal Behavior

While the kinetic theory primarily describes ideal gases, it also helps explain the behavior of real gases under various conditions. Deviations from ideal behavior occur at high pressures and low temperatures, where intermolecular forces and the volume of gas molecules become significant.

• Illustrative Explanation: Think of a can of soda. When you shake it, the pressure inside increases due to the carbon dioxide gas. If you open the can, the gas rapidly escapes, and the behavior of the gas deviates from the ideal gas law due to the interactions between gas molecules and the volume they occupy.

7. Diffusion and Effusion

The kinetic theory of gases explains the processes of diffusion and effusion, which describe how gas molecules spread out and escape through small openings, respectively. The rate of diffusion is influenced by the speed of the gas molecules, which is related to temperature and molecular mass.

• Illustrative Explanation: Imagine a drop of food coloring in a glass of water. Over time, the color spreads throughout the water due to diffusion, as the molecules move randomly and mix. Similarly, gas molecules diffuse through the air, spreading out until they are evenly distributed.

8. Understanding Heat Transfer

The kinetic theory provides insights into heat transfer processes, such as conduction, convection, and radiation. The motion of gas molecules plays a crucial role in transferring thermal energy from one part of a system to another.

• Illustrative Explanation: Consider a pot of water on a stove. As the water at the bottom heats up, the molecules move faster and collide with neighboring molecules, transferring heat throughout the pot. This process continues until the entire pot reaches the same temperature, illustrating how molecular motion facilitates heat transfer.

Conclusion

In conclusion, the kinetic theory of gases is a fundamental framework that explains the behavior of gases in terms of the motion of their molecules. By understanding its principles, assumptions, mathematical formulations, and various applications, we can appreciate the significance of the kinetic theory in explaining gas properties and behaviors. From the ideal gas law to real gas behavior, diffusion, and heat transfer, the kinetic theory of gases provides valuable insights into the microscopic world of gas molecules and their macroscopic effects. As we continue to explore the principles of thermodynamics and molecular motion, the study of the kinetic theory will remain essential for understanding the behavior of gases in various scientific and engineering contexts.