Stress in Physics: A Comprehensive Overview

In the realm of physics, “stress” refers to a measure of the internal forces that develop within a material when it is subjected to external loads or forces. This concept is fundamental in the study of mechanics, materials science, and engineering, as it helps us understand how materials deform, fail, and behave under various conditions. This article will explore the definition of stress, its types, the relationship between stress and strain, the factors affecting stress, and its applications in real-world scenarios, providing illustrative explanations to clarify each concept.

What is Stress?

Definition

In physics, stress is defined as the force applied per unit area within materials. It quantifies the internal resistance of a material to deformation when subjected to external forces. Mathematically, stress ( $\sigma$ ) is expressed as:

$\sigma = \frac{F}{A}$

where:

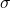$\sigma$ is the stress,
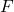$F$ is the applied force,
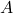$A$ is the cross-sectional area over which the force is applied.
Illustrative Explanation: Imagine pressing down on a soft sponge with your hand. The force you apply creates an internal resistance within the sponge, which is the stress. The larger the area of your hand, the less stress is felt by the sponge for the same amount of force. This illustrates how stress is dependent on both the force applied and the area over which it acts.

Historical Context

The concept of stress in physics has its roots in the study of materials and structures, with significant contributions from early scientists and engineers. Notably, the work of Sir Isaac Newton and later developments by scientists such as Augustin-Louis Cauchy laid the groundwork for understanding stress and strain in materials. Cauchy introduced the concept of stress as a tensor quantity, which is essential for analyzing complex loading conditions in three dimensions.

Illustrative Explanation: Think of the history of stress in physics as a timeline of discovery. Each scientist represents a milestone, contributing to our understanding of how materials respond to forces, much like a series of stepping stones leading to a deeper comprehension of material behavior.

Types of Stress

Stress can be classified into several types based on the nature of the forces acting on a material. The primary types of stress include:

1. Tensile Stress

Tensile stress occurs when a material is subjected to a pulling or stretching force. This type of stress tends to elongate the material and is calculated as:

$\sigma_t = \frac{F_t}{A}$

where $F_t$ is the tensile force.

Illustrative Explanation: Imagine a rubber band being stretched between your fingers. As you pull the ends apart, the rubber band experiences tensile stress, which causes it to elongate. The more you pull, the greater the tensile stress.

2. Compressive Stress

Compressive stress occurs when a material is subjected to a pushing or compressing force. This type of stress tends to shorten the material and is calculated similarly:

$\sigma_c = \frac{F_c}{A}$

where $F_c$ is the compressive force.

Illustrative Explanation: Think of a stack of books being pressed down by another book placed on top. The books at the bottom experience compressive stress as they are pushed together, causing them to shorten slightly under the load.

3. Shear Stress

Shear stress occurs when forces are applied parallel to the surface of a material, causing layers of the material to slide past one another. It is calculated as:

$\tau = \frac{F_s}{A}$

where $F_s$ is the shear force.

Illustrative Explanation: Imagine pushing a deck of cards from one side while holding the other side steady. The cards slide over each other, experiencing shear stress. The force you apply causes the layers of cards to shift, illustrating how shear stress operates.

4. Bending Stress

Bending stress occurs when a material is subjected to a bending moment, causing it to experience both tensile and compressive stresses. This type of stress is common in beams and structural elements.

Illustrative Explanation: Think of a diving board. When a person jumps on one end, the board bends, creating tensile stress on the underside (where it stretches) and compressive stress on the top side (where it compresses). This illustrates how bending stress involves a combination of different stress types.

The Relationship Between Stress and Strain

Stress is closely related to strain, which is the measure of deformation experienced by a material in response to stress. Strain ( $\epsilon$ ) is defined as the change in length per unit length and is expressed as:

$\epsilon = \frac{\Delta L}{L_0}$

where:

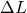$\Delta L$ is the change in length,
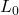$L_0$ is the original length.

Hooke’s Law

For many materials, especially within the elastic limit, the relationship between stress and strain is linear and is described by Hooke’s Law:

$\sigma = E \cdot \epsilon$

where $E$ is the modulus of elasticity, a material property that measures its stiffness.

Illustrative Explanation: Imagine a spring being stretched. When you pull on the spring, it elongates proportionally to the force applied, up to a certain point. This proportionality illustrates Hooke’s Law, where stress and strain are directly related for elastic materials.

Elastic and Plastic Deformation

Elastic Deformation: When a material is subjected to stress within its elastic limit, it will return to its original shape once the stress is removed. This behavior is characterized by elastic deformation.
Plastic Deformation: If the stress exceeds the material’s yield strength, it undergoes plastic deformation, resulting in permanent changes to its shape.
Illustrative Explanation: Think of a clay ball. When you press it gently, it deforms but returns to its original shape when you stop applying pressure (elastic deformation). However, if you press too hard, the clay will change shape permanently (plastic deformation).

Factors Affecting Stress

Several factors can influence the stress experienced by a material, including:

1. Material Properties

Different materials have varying strengths, stiffness, and ductility, which affect how they respond to stress. For example, metals typically exhibit high tensile strength, while rubber is more ductile and can stretch significantly before breaking.

Illustrative Explanation: Imagine two different types of balloons: one made of thick rubber and another made of thin plastic. The thick rubber balloon can withstand more stress before bursting compared to the thin plastic balloon, illustrating how material properties affect stress tolerance.

2. Geometry of the Material

The shape and size of a material can influence how stress is distributed. For instance, a beam with a larger cross-sectional area can distribute the same load over a greater area, resulting in lower stress.

Illustrative Explanation: Think of a wide, flat board versus a narrow stick. If you apply the same weight to both, the board will experience less stress because the weight is spread over a larger area, while the stick will experience higher stress due to its smaller cross-section.

3. Loading Conditions

The manner in which forces are applied to a material can affect the type and magnitude of stress experienced. For example, a sudden impact can create higher stress concentrations than a gradual load.

Illustrative Explanation: Imagine dropping a heavy ball onto a thin sheet of ice. The sudden impact creates a high-stress concentration at the point of contact, potentially causing the ice to crack. In contrast, if you gently place the ball on the ice, the stress is distributed more evenly, reducing the risk of failure.

Applications of Stress in Physics

Understanding stress is crucial in various fields, including engineering, construction, and materials science. Some key applications include:

1. Structural Engineering

In structural engineering, stress analysis is essential for designing safe and efficient structures, such as bridges, buildings, and dams. Engineers must ensure that materials can withstand the stresses imposed by loads, environmental factors, and potential hazards.

Illustrative Explanation: Think of a bridge as a giant balancing act. Engineers must calculate the stresses on different parts of the bridge to ensure it can support the weight of vehicles and withstand wind and other forces, much like a tightrope walker carefully balancing to avoid falling.

2. Material Selection

In materials science, understanding stress helps engineers select appropriate materials for specific applications. Materials with high tensile strength may be chosen for load-bearing components, while more ductile materials may be used in applications requiring flexibility.

Illustrative Explanation: Imagine a chef selecting ingredients for a recipe. Just as the chef chooses the right ingredients based on the dish’s requirements, engineers select materials based on their stress tolerance and properties to ensure optimal performance.

3. Failure Analysis

Stress analysis is critical in failure analysis, where engineers investigate the causes of material failure. By understanding the stresses that led to failure, engineers can improve designs and prevent future incidents.

Illustrative Explanation: Think of a detective examining a crime scene. The detective looks for clues to understand what happened. Similarly, engineers analyze failed components to identify stress factors that contributed to the failure, allowing them to make informed improvements.

4. Biomechanics

In biomechanics, understanding stress is essential for analyzing the forces acting on biological tissues, such as bones and muscles. This knowledge helps in designing medical implants, prosthetics, and understanding injury mechanisms.

Illustrative Explanation: Imagine a tree bending in the wind. Just as the tree experiences stress from the wind, our bones and muscles experience stress from physical activities. Understanding these stresses helps medical professionals design better treatments and devices for patients.

Conclusion

Stress in physics is a fundamental concept that describes the internal forces within materials when subjected to external loads. By understanding its definition, types, relationship with strain, factors affecting stress, and applications, we can appreciate the importance of stress analysis in various fields, from engineering to biomechanics. Whether designing safe structures, selecting appropriate materials, or investigating failures, the principles of stress play a crucial role in ensuring the integrity and performance of materials and systems. As we continue to explore the complexities of stress in physics, we gain valuable insights that inform our understanding of the physical world and enhance our ability to innovate and improve technology.

Blog.Pengayaan.Com