Stress and strain are fundamental concepts in the field of mechanics and materials science, essential for understanding how materials deform under various forces. These concepts are crucial for engineers, architects, and scientists as they design structures, analyze material properties, and ensure safety and reliability in various applications. This article aims to provide an exhaustive overview of stress and strain, detailing their definitions, relationships, types, and applications, along with illustrative explanations of each concept.
Understanding Stress
1. Definition of Stress
Stress is defined as the internal resistance offered by a material to deformation when subjected to an external force. It is quantified as the force applied per unit area and is typically measured in pascals (Pa) or pounds per square inch (psi). The formula for calculating stress (
) is given by:
![\[ \sigma = \frac{F}{A} \]](https://pengayaan.com/blog/wp-content/uploads/2024/12/quicklatex.com-8a85d53d58b1fb497ea32d3075a3115f_l3.png "Rendered by QuickLaTeX.com")
Where:
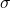- is the stress,
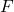is the applied force,
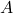is the cross-sectional area over which the force is applied.- Illustrative Explanation: Imagine pressing down on a soft sponge with your hand. The force you apply (your hand) creates an internal resistance within the sponge. Stress measures how much force is applied relative to the area of the sponge that your hand covers. If you press harder (increase the force) or cover a smaller area (like using just a finger), the stress on the sponge increases.
2. Types of Stress
Stress can be categorized into several types based on the nature of the forces acting on the material:
a. Tensile Stress
Tensile stress occurs when a material is subjected to a pulling force, causing it to elongate. It is calculated using the same formula as stress, where the force acts to stretch the material.
- Illustrative Explanation: Think of a rubber band being stretched between two fingers. As you pull the fingers apart, the rubber band experiences tensile stress, causing it to elongate.
b. Compressive Stress
Compressive stress occurs when a material is subjected to a pushing force, causing it to shorten or compress. Like tensile stress, it is calculated using the same formula.
- Illustrative Explanation: Imagine squeezing a thick book between your hands. The force you apply compresses the book, creating compressive stress within its pages.
c. Shear Stress
Shear stress occurs when forces are applied parallel to the surface of a material, causing layers of the material to slide past one another. It is calculated as:
![\[ \tau = \frac{F}{A} \]](https://pengayaan.com/blog/wp-content/uploads/2024/12/quicklatex.com-4a4325fe16d002608484e802dec1903d_l3.png "Rendered by QuickLaTeX.com")
Where 
is the shear stress.
- Illustrative Explanation: Picture a deck of cards. If you push the top card sideways while holding the bottom card still, the top card slides over the bottom card. The force you apply creates shear stress between the cards.
Understanding Strain
3. Definition of Strain
Strain is defined as the measure of deformation representing the displacement between particles in a material body. It is a dimensionless quantity that describes how much a material deforms in response to stress. The formula for calculating strain (
) is given by:
![\[ \epsilon = \frac{\Delta L}{L_0} \]](https://pengayaan.com/blog/wp-content/uploads/2024/12/quicklatex.com-08685f1e5116a5453794ea8c17f14ed5_l3.png "Rendered by QuickLaTeX.com")
Where:
- is the strain,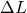
is the change in length,
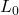is the original length of the material.- Illustrative Explanation: Imagine a rubber band again. If you stretch it from its original length of 10 cm to 12 cm, the change in length (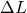) is 2 cm. The strain is the change in length divided by the original length, which in this case would be
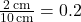or 20%. This means the rubber band has been stretched by 20% of its original length.
4. Types of Strain
Strain can also be categorized into different types based on the nature of deformation:
a. Tensile Strain
Tensile strain occurs when a material is stretched, resulting in an increase in length. It is calculated using the formula for strain.
- Illustrative Explanation: Continuing with the rubber band example, when you stretch it, the tensile strain quantifies how much longer the rubber band has become relative to its original length.
b. Compressive Strain
Compressive strain occurs when a material is compressed, resulting in a decrease in length. It is also calculated using the same formula for strain.
- Illustrative Explanation: If you compress a sponge, the compressive strain measures how much shorter the sponge becomes compared to its original length.
c. Shear Strain
Shear strain occurs when a material is subjected to shear stress, resulting in a change in shape without a change in volume. It is defined as the ratio of the displacement of one layer of the material to the distance between the layers.
- Illustrative Explanation: Returning to the deck of cards, when you slide the top card over the bottom card, the shear strain measures how much the top card has moved relative to the distance between the cards.
Relationship Between Stress and Strain
5. Hooke’s Law
The relationship between stress and strain in elastic materials is described by Hooke’s Law, which states that the stress applied to a material is directly proportional to the strain produced, as long as the material remains within its elastic limit. Mathematically, it can be expressed as:
![\[ \sigma = E \cdot \epsilon \]](https://pengayaan.com/blog/wp-content/uploads/2024/12/quicklatex.com-2a46e775eca9f1d9966b4e2418a2544c_l3.png "Rendered by QuickLaTeX.com")
Where:
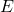is the modulus of elasticity (a material property that measures its stiffness).- Illustrative Explanation: Imagine a spring. When you pull on the spring (applying stress), it stretches (producing strain). Hooke’s Law tells us that the amount the spring stretches is proportional to the force you apply, as long as you don’t stretch it too far (beyond its elastic limit).
6. Elastic Limit and Plastic Deformation
Every material has an elastic limit, which is the maximum stress that can be applied without causing permanent deformation. If the stress exceeds this limit, the material will undergo plastic deformation, meaning it will not return to its original shape when the stress is removed.
- Illustrative Explanation: Think of a piece of clay. If you press it gently, it will deform but return to its original shape when you stop pressing (elastic behavior). However, if you press too hard, the clay will change shape permanently (plastic deformation).
Applications of Stress and Strain
7. Engineering and Construction
Understanding stress and strain is crucial in engineering and construction. Engineers must ensure that structures can withstand the forces they encounter without failing. This involves calculating the stress and strain on materials used in buildings, bridges, and other structures.
- Illustrative Explanation: Consider a bridge. Engineers analyze the stress on the bridge’s beams to ensure they can support the weight of vehicles without bending or breaking. They also consider how the materials will strain under load to prevent structural failure.
8. Material Testing
Stress and strain are fundamental in material testing, where materials are subjected to controlled forces to determine their mechanical properties, such as tensile strength, compressive strength, and ductility.
- Illustrative Explanation: Imagine a laboratory where scientists test the strength of different materials. They apply forces to samples and measure how much they stretch or compress, helping them understand which materials are best for specific applications.
9. Biomechanics
In biomechanics, stress and strain are used to analyze the forces acting on biological tissues, such as bones, muscles, and tendons. Understanding how these tissues respond to stress is essential for designing medical devices and rehabilitation programs.
- Illustrative Explanation: Think of a runner’s knee. Researchers study the stress and strain on the knee joint during running to understand how to prevent injuries and improve performance.
10. Geotechnical Engineering
In geotechnical engineering, stress and strain are critical for analyzing soil behavior under loads, such as buildings or roads. Engineers must understand how soil will deform under pressure to ensure stability and prevent settlement.
- Illustrative Explanation: Picture a tall building being constructed on soft soil. Engineers must calculate the stress the building will exert on the soil and how much the soil will strain to ensure the building remains stable and does not sink.
Conclusion
In conclusion, stress and strain are fundamental concepts in mechanics and materials science that describe how materials respond to external forces. By exploring their definitions, types, relationships, and applications, we can appreciate the significance of stress and strain in various fields, including engineering, construction, biomechanics, and geotechnical engineering. Understanding these concepts not only enhances our knowledge of material behavior but also provides valuable insights into the design and analysis of structures and systems. As technology continues to advance, the study of stress and strain will remain essential for ensuring safety, reliability, and performance in a wide range of applications.