Current density is a fundamental concept in electromagnetism and electrical engineering that describes the distribution of electric current in a given area. It is a crucial parameter for understanding how electric currents flow through conductors, semiconductors, and other materials. This article will provide a detailed exploration of current density, including its definition, mathematical formulation, physical significance, applications, and illustrative explanations to enhance understanding.
1. Definition of Current Density
Current density (
) is defined as the amount of electric current (
) flowing per unit area (
) of a cross-section through which the current flows. It is a vector quantity, meaning it has both magnitude and direction. The mathematical expression for current density is given by:
![\[ \mathbf{J} = \frac{I}{A} \]](https://pengayaan.com/blog/wp-content/uploads/2024/12/quicklatex.com-80b5591f663ce079ae787be9ac9b8afa_l3.png "Rendered by QuickLaTeX.com")
Where:
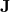- is the current density (measured in amperes per square meter, A/m²).
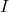- is the electric current (measured in amperes, A).
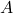- is the cross-sectional area through which the current flows (measured in square meters, m²).
Illustrative Explanation
Imagine a garden hose through which water is flowing. If you measure the amount of water flowing through the hose, that represents the current (). Now, if you consider the diameter of the hose as the cross-sectional area (), the current density () would represent how much water is flowing through each square meter of the hose’s cross-section. A wider hose would have a lower current density for the same flow rate, while a narrower hose would have a higher current density.
2. Mathematical Representation of Current Density
Current density can be expressed in different forms depending on the context, particularly in relation to the direction of current flow and the geometry of the conductor.
2.1. Uniform Current Density
In cases where the current density is uniform across a cross-section, the current density can be expressed as:
![\[ \mathbf{J} = J \hat{n} \]](https://pengayaan.com/blog/wp-content/uploads/2024/12/quicklatex.com-626649f61d65a48f133a735936c7aa2c_l3.png "Rendered by QuickLaTeX.com")
Where:
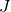is the magnitude of the current density.
is the unit vector indicating the direction of current flow.
2.2. Non-Uniform Current Density
In situations where the current density varies across a cross-section, it can be expressed as a function of position:
![\[ \mathbf{J} = J(x, y, z) \hat{n} \]](https://pengayaan.com/blog/wp-content/uploads/2024/12/quicklatex.com-e0ae38fecad77bdf7589a96427e9272d_l3.png "Rendered by QuickLaTeX.com")
Where 
represents the current density at specific coordinates in three-dimensional space.
Illustrative Explanation
Consider a river flowing through a narrow canyon. If the river flows uniformly, the current density is consistent throughout the width of the canyon. However, if the river narrows at one point, the current density increases in that section, similar to how a garden hose’s flow rate increases when pinched. In this analogy, the river’s flow represents the electric current, while the canyon’s width represents the cross-sectional area.
3. Physical Significance of Current Density
Current density is significant for several reasons:
3.1. Understanding Current Flow
Current density provides insight into how electric current flows through different materials. It helps engineers and scientists analyze the behavior of conductors, semiconductors, and insulators under various conditions.
3.2. Heat Generation
As current flows through a conductor, it generates heat due to resistance. The amount of heat generated is proportional to the square of the current density, as described by Joule’s law:
![\[ P = I^2 R \]](https://pengayaan.com/blog/wp-content/uploads/2024/12/quicklatex.com-a8e8abc4462800cb7750de707b678c23_l3.png "Rendered by QuickLaTeX.com")
Where 
is the power (heat) generated, is the current, and 
is the resistance. Higher current densities can lead to increased heating, which is critical in designing electrical components.
3.3. Electromagnetic Fields
Current density is related to the generation of magnetic fields through Ampère’s Law, which states that the magnetic field (
) around a conductor is proportional to the current density:
![\[ \nabla \times \mathbf{B} = \mu_0 \mathbf{J} \]](https://pengayaan.com/blog/wp-content/uploads/2024/12/quicklatex.com-d0b8ea1ffa0cdc3199a724ee62771f5c_l3.png "Rendered by QuickLaTeX.com")
Where 
is the permeability of free space. This relationship is fundamental in understanding electromagnetism and the operation of devices like inductors and transformers.
Illustrative Explanation
Think of current density as the flow of traffic on a highway. A higher current density corresponds to more cars (current) traveling through a specific lane (cross-sectional area). If too many cars are in one lane, traffic jams (heat generation) occur, leading to delays (potential damage to the road). Similarly, in electrical systems, high current densities can lead to overheating and potential failure of components.
4. Applications of Current Density
Current density has numerous applications across various fields, including:
4.1. Electrical Engineering
In electrical engineering, current density is crucial for designing circuits, ensuring that components can handle the expected current without overheating or failing. Engineers use current density calculations to select appropriate wire gauges and materials.
4.2. Semiconductor Devices
In semiconductor physics, current density is essential for understanding the behavior of diodes, transistors, and integrated circuits. It helps in analyzing how charge carriers (electrons and holes) move through semiconductor materials.
4.3. Electromagnetic Devices
Current density plays a vital role in the design of electromagnetic devices, such as motors, generators, and transformers. Understanding how current density affects magnetic fields is crucial for optimizing the performance of these devices.
4.4. Energy Transmission
In power transmission systems, current density is a key factor in determining the efficiency of energy transfer. High current densities can lead to energy losses due to resistive heating, making it essential to optimize conductor sizes and materials.
Illustrative Explanation
Consider a power plant generating electricity. The current density in the transmission lines must be carefully managed to ensure efficient energy transfer. If the current density is too high, it can lead to energy losses (like a leaky pipe), resulting in wasted resources. Engineers must balance the current density to maximize efficiency while preventing overheating and ensuring safety.
5. Factors Affecting Current Density
Several factors can influence current density in a conductor:
5.1. Material Properties
The electrical conductivity of a material affects current density. Conductors like copper and aluminum have high conductivity, allowing for higher current densities compared to insulators like rubber or glass.
5.2. Temperature
Temperature can significantly impact current density. As temperature increases, the resistance of most conductors also increases, which can lead to a decrease in current density for a given voltage.
5.3. Cross-Sectional Area
The size of the conductor’s cross-sectional area directly affects current density. A larger area allows for a lower current density for the same amount of current, reducing the risk of overheating.
Illustrative Explanation
Imagine a water pipe carrying water. If the pipe is made of a material that easily allows water to flow (like copper), it can handle a higher flow rate (current density). If the pipe heats up (temperature increase), it may become less efficient, similar to how a conductor’s resistance increases with temperature. If the pipe’s diameter is increased, the flow rate can be maintained without increasing the pressure (current density), preventing potential leaks (overheating).
6. Conclusion
In conclusion, current density is a fundamental concept in electromagnetism and electrical engineering that describes the distribution of electric current in a given area. Understanding current density, its mathematical representation, physical significance, applications, and the factors affecting it is crucial for analyzing and designing electrical systems. By grasping the concept of current density, engineers and scientists can optimize the performance of electrical devices, ensure safety, and enhance efficiency in energy transmission. As technology continues to advance, the importance of current density will remain central to innovations in electronics, power systems, and electromagnetism, paving the way for a more efficient and sustainable future. Through careful consideration of current density, we can harness the power of electricity effectively and responsibly.